Various laws of movement learning and control have been proposed on the basis of research. In this entry, the focus is on three of the most firmly established of these laws: the law of practice, Fitts’s law, and Hick’s law. These laws are of interest to sport and exercise psychologists because they specify relatively simple relationships between different variables related to movement learning and control. The defining feature of these laws is that the relationships they specify apply to many different populations (e.g., males and females, the young and the elderly) and types of movement in sport and exercise. The laws are useful because they afford the identification of practice-related conditions that promote movement learning and predictions about how individuals will perform in various situations requiring movement. As such, the laws provide a basis for the design of practice and training curricula, schedules, and environments and also for the design of equipment for sport and exercise. The laws are described here, in turn.
The Law of Practice
The law of practice states that more practice of a motor task will lead to more learning of that task. This definition is usually accompanied by a second statement: Changes in motor task performance that follow practice are generally large and rapid at first and become gradually smaller with continued practice. As an example, consider a scenario in which 30 novice gymnasts are each provided with 10 practice sessions, in which the gymnast attempts to stand one-footed on the beam, with the other leg bent at the knee, without wobbling or falling off the beam. Each practice session is separated by a 3 days’ rest, lasts 2 hours, and begins with one test of how long, in seconds, the gymnast can do the task before they experience an obvious wobble or a fall. Consider then that the average of the 30 gymnasts’ test scores is calculated for each of the 10 days’ tests and the average values are plotted on a graph against the practice sessions undertaken. The law of practice predicts that the graph would look something like the (hypothetical) example graph shown in Figure 1. Note from the graph that, consistent with the law of practice, the increase in time spent balanced one-footed on the beam is greatest early in practice, between Practice Sessions 1 and 2, and becomes smaller with more practice; the smallest increase occurs between Practice Sessions 9 and 10. One interpretation of this effect is that the rate of improvement at any point in practice is determined by how much learning remains. Early in the practice of a given task, much remains to be learned and thus the rate of learning is very rapid. After extensive practice, there is not much left to be learned, so learning is much slower. At this late stage of learning, it can take months or even years of practice before any noticeable gain in performance is attained. As with all of the laws described here, the law of practice applies to many different populations and types of are often practiced in isolation from other components of the sport, which affords an increase in the rate of attempts to practice the component; a tennis player might devote an entire practice session to practicing her backhand volley.
Academic Writing, Editing, Proofreading, And Problem Solving Services
Get 10% OFF with 24START discount code
Thus, the deliberate practice of a task contrasts with mere engagement in, and experience of, a task. A common example of this difference concerns golf. A golfer seeking to improve his game adds a nine-hole round with friends to his weekly golf schedule, which he considers extra practice but no improvement in his game results. On closer inspection, however, the holes are played in a relaxed way and the “practice” session lacks the qualities of deliberate practice. Thus, this golfer’s addition of nine holes to his weekly golf schedulemovement task, from turns in skiing to throws in discus.
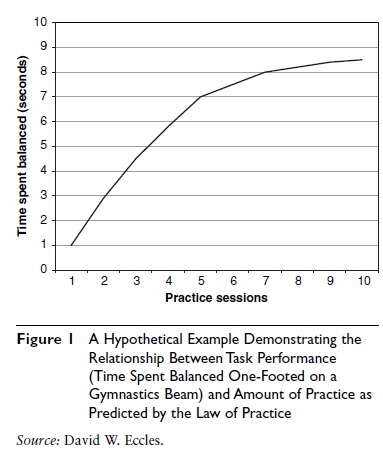
Figure 1 A Hypothetical Example Demonstrating the Relationship Between Task Performance (Time Spent Balanced One-Footed on a Gymnastics Beam) and Amount of Practice as Predicted by the Law of Practice
It is important to define practice in relation to the law of practice. Practice activities can differ widely, and these differences are not always appreciated, leading some individuals to engage in what they consider to be practice activities only to be disappointed when little or no learning follows. While the exact qualities of practice activities affording optimal learning in sport and exercise are in contention, Anders Ericsson and his colleagues have proposed the term deliberate practice to capture some of the qualities that appear associated with learning. Deliberate practice is structured, purposeful practice relevant to improving performance. It requires concentration and/ or effort and is often undertaken alone to allow the learner to concentrate; these characteristics make the process of engaging in deliberate practice inherently unenjoyable, even if the result of deliberate practice is enjoyable, such as the acquisition of a new skill. Deliberate practice activities are often focused on improving weaker components of current performance—for example, a backhand volley in tennis. Coaches are often employed to identify these components and prescribe specific deliberate practice activities to improve them— for example, drills to practice backhand volleys at various distances from the net. These components affords him the opportunity to obtain more experience of the game but does not involve its deliberate practice. Hence, there is no improvement in the golfer’s game.
Fitts’s Law
Fitts’s law describes in more formal terms what is known in everyday terms as the speed-accuracy trade-off; that is, it describes the relationship between the speed of a movement and its accuracy. In the 1950s, researcher Paul Fitts created an experiment in which two targets were laid upon a table-type surface in front of a participant; one target was positioned slightly to the left of the participant and the other slightly to the right. The participant’s task was simply to tap anywhere on the surface of the left target and then the right target using a stylus, which was a penlike rod, held in one hand, and then repeat this (i.e., left target, right target, left target) as fast as possible for a specific time such as 30 seconds. The task was like hitting one drum with a drumstick, and then another drum (with the same drumstick in the same hand), then the first drum, and so on, as fast as possible. In the experiment, the width of the two targets could be varied; for example, they each might be small, at 2 cm in width, or large, at 10 cm in width. Also, the distance between the targets could be varied; for example, they might be closely spaced, with 10 cm between them, or farther apart, with 20 cm between them. The task was scored as the number of taps the participant could make in the time allowed. Accuracy was stressed by asking the participant to make sure that no more than 5% of the taps fell outside of a target. Fitts calculated the average time taken between two consecutive taps by dividing the time over which the participant was requested to tap (e.g., 30 seconds) by the number of taps made: If30 taps were made in 30 seconds, the average time between 2 consecutive taps was 1 second. In other words, it took the participant a second on average to tap one target and then move his or her hand to the other target and tap that target. Fitts called this variable movement time (MT).
Fitts explored what happened to MT when the width of each target and the distance between the targets was varied. When targets at a fixed distance apart (e.g., 10 cm) each had their width reduced (e.g., from 4 cm to 2 cm), MT increased; participants appeared to need to move slower to be more accurate in the spatial positioning of their taps. Also, when targets of a fixed width (e.g., 4 cm) were moved farther apart (e.g., from 10 cm to20 cm), MT slowed. Participants appeared to need to take longer to move their hand over the extra distance if they were to maintain the spatial accuracy of their tapping. While these findings were interesting, Fitts’s really important finding was that MT increased by a constant amount whenever the distance between the targets was doubled or the size of each target was halved. Furthermore, this finding was basically the same for all the participants tested. This meant that Fitts was able to predict, with reasonable accuracy, the MT of participants he had not yet tested, based simply on the width of the targets and their distance apart. Of course, not every task involving movement is like Fitts’s tapping task, but since his research, studies involving different populations and different types of movement task have yielded findings consistent with his own. These consistent findings have led to Fitts’s early observations being considered a law of motor control.
One explanation for Fitts’s law, in simple terms, is as follows. An increase in the speed with which a given limb (e.g., an arm) is moved requires an increase in the impulse applied by the muscles to that limb; note that an impulse is the amount of force applied multiplied by the time for which that force is applied. A characteristic of the human movement system is that there is a slight and unavoidable difference between the impulse an individual intends to apply to a limb to make it move and the impulse that the individual actually applies to the limb. This difference can be considered error. Research has indicated that the larger the impulse applied, the greater the error. Now let us put these separate statements together: When humans attempt to move a limb faster, they must apply a larger impulse to the limb: The larger the impulse applied to the limb, the larger the error in the impulse applied; the larger the error in the impulse applied, the greater the inaccuracy in the spatial positioning of the limb.
While tasks involving simple movements like Fitts’s tapping task offer the researcher a chance to study motor control under controlled laboratory conditions, these movements might seem at first glance to be of limited relevance to sport and exercise. However, simple movements form the basis for the more complicated movements made in sport and exercise and, as such, Fitts’s law is reflected in sport and exercise-related movements. For example, pole-vaulters take a long time to learn to place the pole tip into the box (i.e., the hole in the ground) correctly at speed; until then, they use a shorter approach, which affords a relatively slow approach run, to achieve an accurate tip placement. In addition, rock climbers who attempt to reach up very quickly to grab the next hold, because they feel their other points of contact with the rock are tenuous, are less likely to accurately place their fingers on the desired hold.
Hick’s Law
Hick’s law describes the relationship between the time taken to prepare a movement response and the number of possible movement response alternatives. In more everyday terms, the law states that individuals are slower to react when required if it is not clear before they are required to react exactly how they should react—that is, if it is not clear what kind of movement response is required. An example of Hick’s law can be seen in football. Consider a down in which the defense is unclear about what type of offensive play the opponent is likely to run: When the ball is snapped, the defense will be relatively slow to react with an appropriate response. In contrast, if the defense knows exactly what offensive play the opponent is going to run, the defense will be relatively fast to react with an appropriate response when the ball is snapped.
In the 1950s, researchers William Edmund Hick and Ray Hyman studied reaction time (RT) using what has become known as a “choice reaction time” task. In one type of this task, the participant is seated, with one hand laying palm-down on a “response panel,” and each of the four fingers of the hand resting on a separate button mounted in the panel. In front of the participant, four lights are presented in a row (i.e., horizontally) at head height. Each light is considered a stimulus, to which the participant must respond as fast as possible with a movement. Specifically, when a light is lit, the participant must press the button, from the four available, that corresponds to the lit light. Thus, if the leftmost light is lit, she must press the leftmost button with her index finger (if she is right-handed), whereas if the rightmost light is lit, she must press the rightmost button with her little finger, and so on. Usually, participants are unable to predict exactly which light will be lit or when it will be lit and thus are unable to initiate a response in advance of a light being lit. RT is measured as the time taken from a light being lit to the beginning of the participant’s finger movement on the button.
In the choice RT task presented previously, there were four “stimulus–response” (S–R) choices or alternatives; that is, the participant could be presented with one of four stimuli, and each of these stimuli required a unique response. For example, when the second-to-left light was lit, a press of the second-to-left button was required by the middle finger. Hick and Hyman studied the relationship between RT and the number of S–R alternatives by presenting various numbers of lights associated with an equal number of buttons to be pressed. One study condition presented to the participant might feature two S–R alternatives, involving two lights and two buttons, whereas the next study condition presented might involve four S–R alternatives, as in the previous example. The researchers found that RT slowed as the number of S–R alternatives increased. In other words, when it was less clear how they would be required to respond, participants were slower to react.
However, Hick and Hyman’s findings extended beyond identifying that RT slowed under these circumstances. What these researchers discovered was that RT slowed by a constant amount, which was approximately 150 milliseconds on average for the type of choice RT task described here, every time the number of S–R alternatives was doubled. Of course, RT was also quickened by this amount every time the number of S–R alternatives was halved. Thus, when a “one light and one button condition” was changed to a “two lights and two buttons” condition, RT slowed by 150 milliseconds on average, and when an “eight lights and eight buttons” condition was changed to a “two lights and two buttons” condition, RT quickened by 300 milliseconds on average.
Researchers have found that Hick and Hyman’s findings apply to different populations and different types of movement task, which has led to the finding being considered a law of motor control. One explanation for Hick’s law, in simple terms, is that, when there are more S–R alternatives, the participant must process (i.e., think about) more information to be able to identify and produce the appropriate response. As humans can only process a limited amount of information in a given time, RT is slower when there is more information to be processed.
As with Fitts’s law, the choice RT task described here might seem at first glance to be of limited relevance to movements made in sport and exercise. However, Hick’s law helps explain performance in real sports, especially sports in which responding faster conveys a performance advantage. Consider a defensive team in football that knows the opponent’s offense has two key running plays and two key passing plays and then observes the opponent’s only ball carrier get injured so that running plays are no longer an option. At the line of scrimmage, the defense knows there are now only two possible play options, not four. In terms of Hick’s law, the number of S–R alternatives has been halved, affording the defense a quicker RT (by 150 milliseconds) at the snap. Hick’s law is also reflected in findings from studies of skilled athletes’ preparation for upcoming competitions. For example, David Eccles and his colleagues presented evidence that skilled athletes gather information affording the identification of (and thus a reduction in uncertainty about) the types of stimuli that will be presented during a competition. An example of this process is when athletes and coaches in soccer or football watch game film of an upcoming opponent to identify patterns of play unique to that opponent or when kayakers and orienteers explore the river and forest, respectively, in which they will compete (under time pressure) to identify in advance the demands unique to these environments. According to Hick’s law, these information gathering activities, which reduce uncertainty about the types of stimuli that will be presented during a competition, lead to quicker RTs during that competition.
Conclusion
In conclusion, there are three well-established laws of movement learning and control: the law of practice, Fitts’s law, and Hick’s law. The law of practice describes the relationship between practice and learning; Fitts’s law describes the relationship between movement speed and accuracy; and Hick’s law describes the relationship between the time taken to prepare a movement response and the number of possible movement response alternatives. The relationships described by these laws apply to many different populations and types of movement in sport and exercise. The laws afford the identification of practice-related conditions that promote movement learning and predictions about how individuals will perform in situations requiring movement. As such, the laws are fundamental within the discipline of sport and exercise psychology (SEP).
References:
- Eccles, D. W., Ward, P., & Woodman, T. (2009). The role of competition-specific preparation in expert sport performance. Psychology of Sport and Exercise, 10,96–107.
- Ericsson, K. A., Krampe, R. Th., & Tesch-Römer, C. (1993). The role of deliberate practice in the acquisition of expert performance. Psychological Review, 100, 363–406.
- Lee, T. D. (2011). Motor control in everyday actions.Champaign, IL: Human Kinetics.
- Magill, R. A. (2007). Motor learning and control: Concepts and applications. New York: McGraw-Hill.
- Schmidt, R. A., & Lee, T. D. (2011). Motor control and learning: A behavioral emphasis (5th ed.). Champaign, IL: Human Kinetics.
See also: